1) Sketch the parabola, and lable the focus, vertex and directrix. a) (y - 1)^2 = -12(x + 4) b) i) y^2 - 6y -2x + 1 = 0, ii) y =
Por um escritor misterioso
Last updated 17 fevereiro 2025

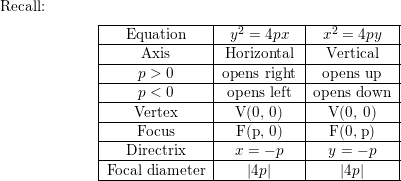
An equation of a parabola is given. (a) Find the focus, dire

SOLVED: Use the given equation to identify the direction the parabola is opening, and the vertex, focus, and directrix for the parabola. Then, graph the parabola. Include the focus, vertex, directrix, and
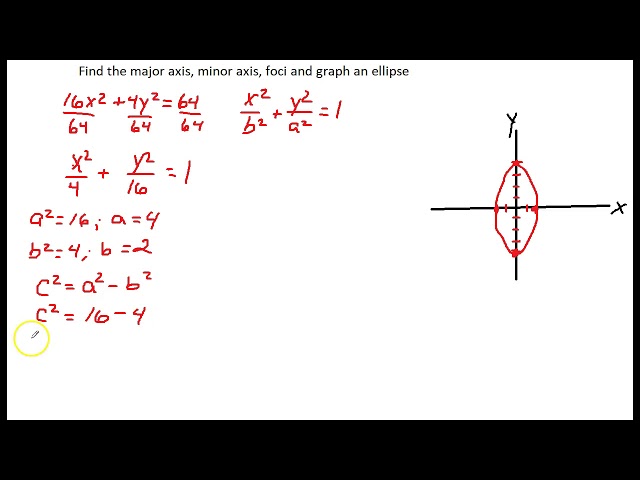
Find the major axis, minor axis, foci and graph an ellipse
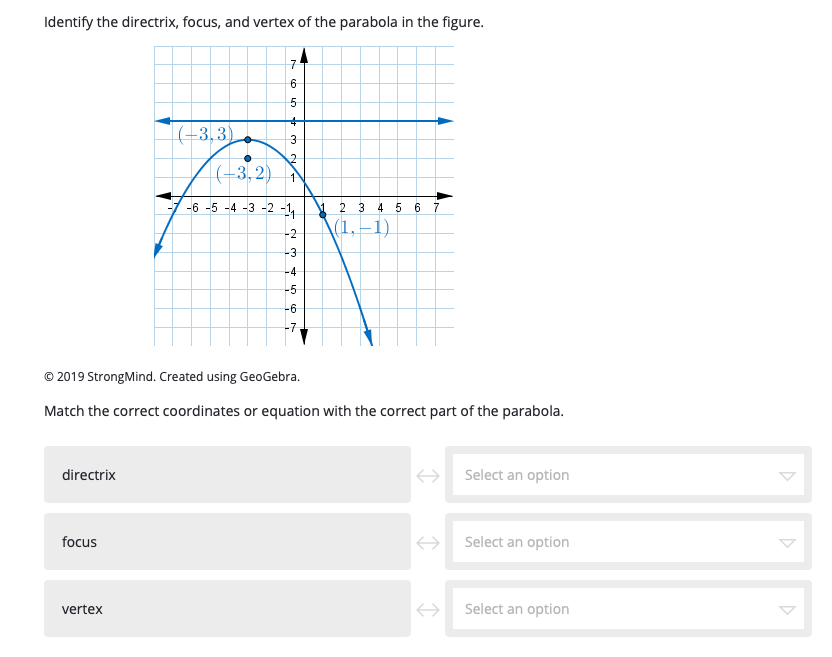
Answered: Identify the directrix, focus, and…
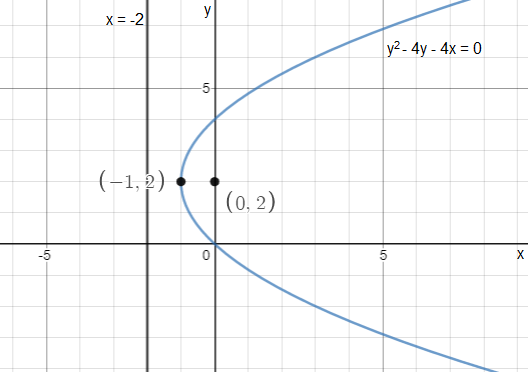
Find the vertex, focus, and directrix of the parabola, and s
How to tell if a hyperbola opens up or down - Quora

Solved 1. Use the definition of the parabola to write an
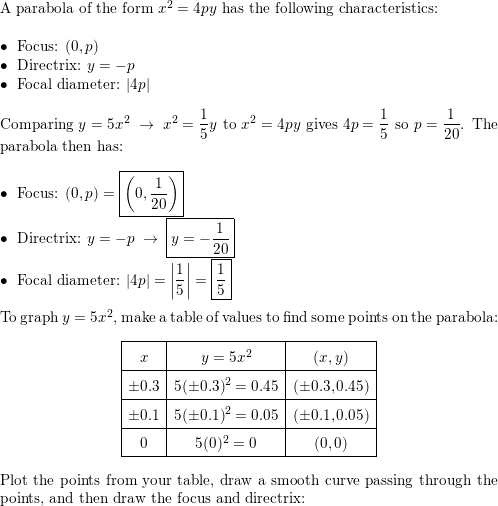
Find the focus, directrix, and focal diameter of the parabol

4 Parabolas WS #2-1.pdf - Name Parabolas Worksheet #2 Write the equation of each parabola described below. 1. Vertex -2 3 and focus 4 3 3. Focus

Conic sections: Analyzing Conic Sections with the Algebraic Method - FasterCapital
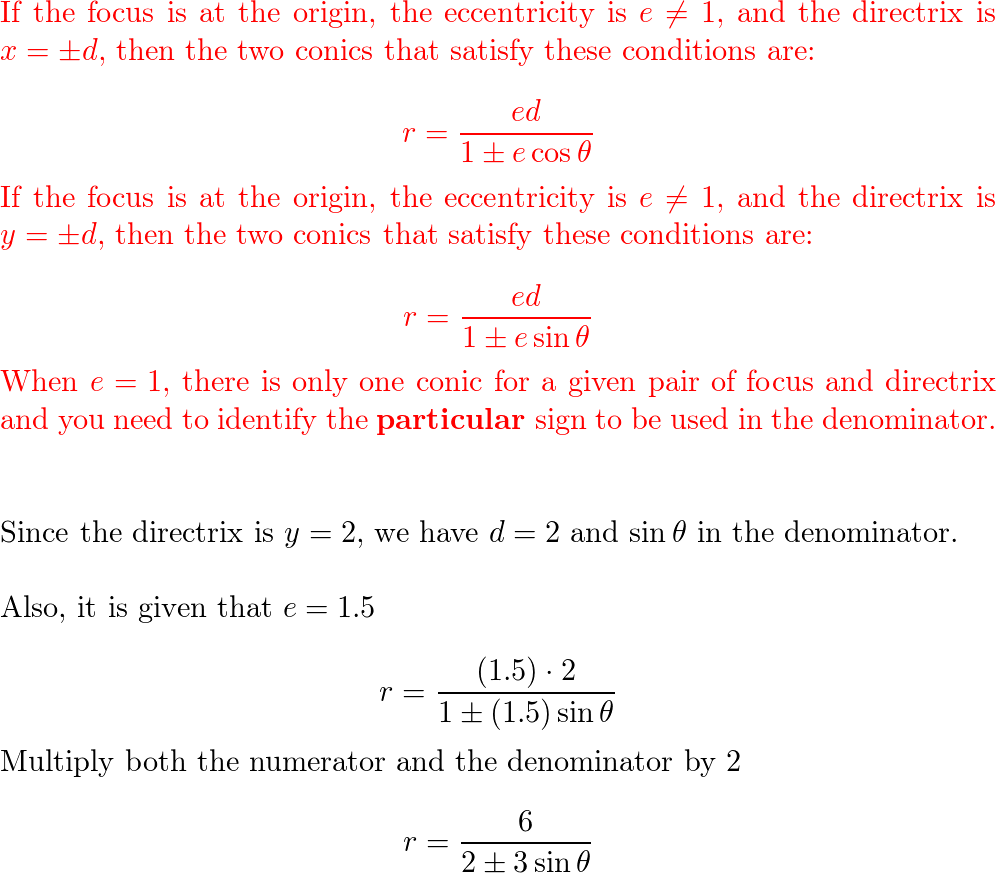
Write a polar equation of a conic with the focus at the orig
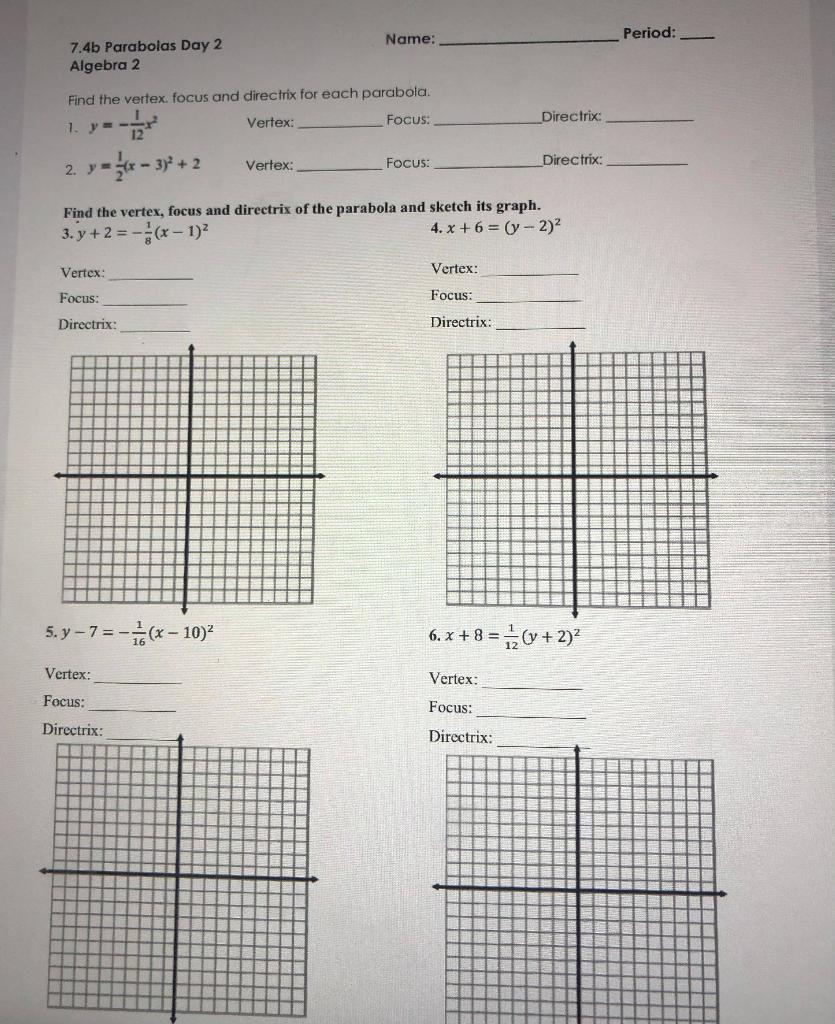
Solved Name: Period: 7.4b Parabolas Day 2 Algebra 2 Find the

How can I calculate properly the graph of a quadratic function? - Mathematics Stack Exchange

Find a polar equation of the conic with its focus at the pole. Parabola; (8, 0)
Recomendado para você
-
Directx 9.0C Compatible 3D Accelerated 64Mb Video Card Download - Colaboratory17 fevereiro 2025
-
 Download DirectX Free - Latest Version 2023 ✓17 fevereiro 2025
Download DirectX Free - Latest Version 2023 ✓17 fevereiro 2025 -
Path Tracer x Directx 1217 fevereiro 2025
-
 pc BILLY HATCHER And The GIANT EGG Game (NI) REGION FREE PC CD-ROM17 fevereiro 2025
pc BILLY HATCHER And The GIANT EGG Game (NI) REGION FREE PC CD-ROM17 fevereiro 2025 -
 AMD DirectX® 12 (DX12) Technology17 fevereiro 2025
AMD DirectX® 12 (DX12) Technology17 fevereiro 2025 -
 Mysterious Journey II : Video Games17 fevereiro 2025
Mysterious Journey II : Video Games17 fevereiro 2025 -
 Xbox MB system memory IBM 3-way symmetric core processor ATI GPU with embedded EDRAM 12x DVD Optional Hard disk. - ppt download17 fevereiro 2025
Xbox MB system memory IBM 3-way symmetric core processor ATI GPU with embedded EDRAM 12x DVD Optional Hard disk. - ppt download17 fevereiro 2025 -
 AMD A8-3850 APU Review - Llano for Desktop - Overclockers17 fevereiro 2025
AMD A8-3850 APU Review - Llano for Desktop - Overclockers17 fevereiro 2025 -
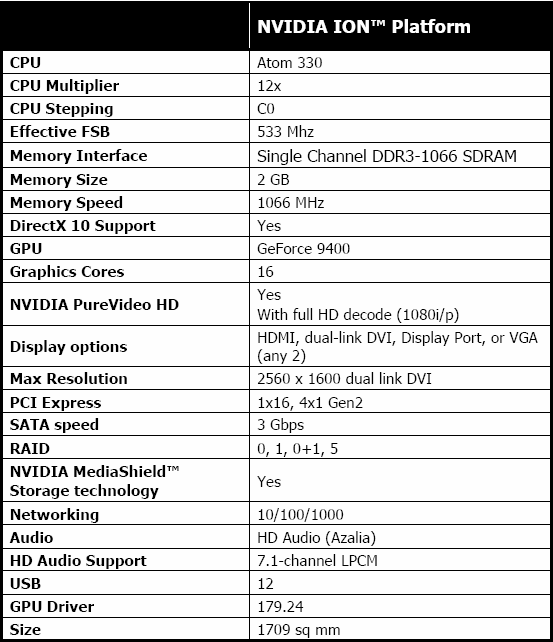 NVIDIA Ion Reference PC Platform Deep Dive17 fevereiro 2025
NVIDIA Ion Reference PC Platform Deep Dive17 fevereiro 2025 -
 Placa de Vídeo JieShuo Radeon RX 6600 XT, 8GB, GDDR6, 128-Bit, Nova ou 12X 144,30 - Computadores e acessórios - Vila Marumby, Maringá 123317347617 fevereiro 2025
Placa de Vídeo JieShuo Radeon RX 6600 XT, 8GB, GDDR6, 128-Bit, Nova ou 12X 144,30 - Computadores e acessórios - Vila Marumby, Maringá 123317347617 fevereiro 2025
você pode gostar
-
 Meninas: a homens não choram homens vendo que outra semana no cartoon acabou: mm Episódio Final I Outra Semana no Cartoon I EOS5 I Cartoon Network - iFunny Brazil17 fevereiro 2025
Meninas: a homens não choram homens vendo que outra semana no cartoon acabou: mm Episódio Final I Outra Semana no Cartoon I EOS5 I Cartoon Network - iFunny Brazil17 fevereiro 2025 -
 DEMON SLAYER TANJIRO KAMADO STATUE, 3D models download17 fevereiro 2025
DEMON SLAYER TANJIRO KAMADO STATUE, 3D models download17 fevereiro 2025 -
 Xbox BUYS Activision - Call Of Duty Overwatch Diablo & MORE What ABOUT PlayStation?17 fevereiro 2025
Xbox BUYS Activision - Call Of Duty Overwatch Diablo & MORE What ABOUT PlayStation?17 fevereiro 2025 -
 GameSir G7 Wired Controller for Xbox Series XS, Xbox One and Windows 10/11 - PC Gaming Gamepad with 3.5mm Audio Jack (2 Swappable Faceplates) : Video Games17 fevereiro 2025
GameSir G7 Wired Controller for Xbox Series XS, Xbox One and Windows 10/11 - PC Gaming Gamepad with 3.5mm Audio Jack (2 Swappable Faceplates) : Video Games17 fevereiro 2025 -
 Assistir Hataraku Saibou (Cells at Work!) Episódio 001 Online17 fevereiro 2025
Assistir Hataraku Saibou (Cells at Work!) Episódio 001 Online17 fevereiro 2025 -
 Rangiku Matsumoto Sexy Bleach 62 by michaelxgamingph on DeviantArt17 fevereiro 2025
Rangiku Matsumoto Sexy Bleach 62 by michaelxgamingph on DeviantArt17 fevereiro 2025 -
 Descubra os códigos secretos para acessar as categorias escondidas da Netflix17 fevereiro 2025
Descubra os códigos secretos para acessar as categorias escondidas da Netflix17 fevereiro 2025 -
 Battlefield 4 Rent-a-Server Now Live17 fevereiro 2025
Battlefield 4 Rent-a-Server Now Live17 fevereiro 2025 -
 Sonic the Hedgehog (Paramount), Sonic Zona Wiki17 fevereiro 2025
Sonic the Hedgehog (Paramount), Sonic Zona Wiki17 fevereiro 2025 -
 Pokemon Pikachu 3 Silver Figures17 fevereiro 2025
Pokemon Pikachu 3 Silver Figures17 fevereiro 2025